We live in a wonderfully complex universe, and we are curious about it by nature. Time and again we have wondered--- why are we here? Where did we and the world come from? What is the world made of? It is our privilege to live in a time when enormous progress has been made towards finding some of the answers. String theory is our most recent attempt to answer the last (and part of the second) question.
So, what is the world made of? Ordinary matter is made of atoms, which are in turn made of just three basic components: electrons whirling around a nucleus composed of neutrons and protons. The electron is a truly fundamental particle (it is one of a family of particles known as leptons), but neutrons and protons are made of smaller particles, known as quarks. Quarks are, as far as we know, truly elementary.
Our current knowledge about the subatomic composition of the universe is summarized in what is known as the Standard Model of particle physics. It describes both the fundamental building blocks out of which the world is made, and the forces through which these blocks interact. There are twelve basic building blocks. Six of these are quarks--- they go by the interesting names of up, down, charm, strange, bottom and top. (A proton, for instance, is made of two up quarks and one down quark.) The other six are leptons--- these include the electron and its two heavier siblings, the muon and the tauon, as well as three neutrinos.
There are four fundamental forces in the universe: gravity, electromagnetism, and the weak and strong nuclear forces. Each of these is produced by fundamental particles that act as carriers of the force. The most familiar of these is the photon, a particle of light, which is the mediator of electromagnetic forces. (This means that, for instance, a magnet attracts a nail because both objects exchange photons.) The graviton is the particle associated with gravity. The strong force is carried by eight particles known as gluons. Finally, the weak force is transmitted by three particles, the W+, the W- , and the Z.
The behavior of all of these particles and forces is described with impeccable precision by the Standard Model, with one notable exception: gravity. For technical reasons, the gravitational force, the most familiar in our every day lives, has proven very difficult to describe microscopically. This has been for many years one of the most important problems in theoretical physics-- to formulate a quantum theory of gravity.
In the last few decades, string theory has emerged as the most promising candidate for a microscopic theory of gravity. And it is infinitely more ambitious than that: it attempts to provide a complete, unified, and consistent description of the fundamental structure of our universe. (For this reason it is sometimes, quite arrogantly, called a 'Theory of Everything').
The essential idea behind string theory is this: all of the different 'fundamental ' particles of the Standard Model are really just different manifestations of one basic object: a string. How can that be? Well, we would ordinarily picture an electron, for instance, as a point with no internal structure. A point cannot do anything but move. But, if string theory is correct, then under an extremely powerful 'microscope' we would realize that the electron is not really a point, but a tiny loop of string. A string can do something aside from moving--- it can oscillate in different ways. If it oscillates a certain way, then from a distance, unable to tell it is really a string, we see an electron. But if it oscillates some other way, well, then we call it a photon, or a quark, or a ... you get the idea. So, if string theory is correct, the entire world is made of strings!
Perhaps the most remarkable thing about string theory is that such a simple idea works--- it is possible to derive (an extension of) the Standard Model (which has been verified experimentally with incredible precision) from a theory of strings. But it should also be said that, to date, there is no direct experimental evidence that string theory itself is the correct description of Nature. This is mostly due to the fact that string theory is still under development. We know bits and pieces of it, but we do not yet see the whole picture, and we are therefore unable to make definite predictions. In recent years many exciting developments have taken place, radically improving our understanding of what the theory is.
Wednesday, October 27, 2010
Wednesday, October 13, 2010
Black Holes
There are many popular myths concerning black holes , many of them perpetuated by Hollywood. Television and movies have portrayed them as time-traveling tunnels to another dimension, cosmic vacuum cleaners sucking up everything in sight, and so on. It can be said that black holes are really just the evolutionary end point of massive star . But somehow, this simple explanation makes them no easier to understand or less mysterious.
Black Holes: What Are They?
Black holes are the evolutionary endpoints of stars at least 10 to 15 times as massive as the Sun. If a star that massive or larger undergoes a supernova explosion, it may leave behind a fairly massive burned out stellar remnant. With no outward forces to oppose gravitational forces, the remnant will collapse in on itself. The star eventually collapses to the point of zero volume and infinite density, creating what is known as a " singularity ". Around the singularity is a region where the force of gravity is so strong that not even light can escape. Thus, no information can reach us from this region. It is therefore called a black hole, and its surface is called the " event horizon ". But contrary to popular myth, a black hole is not a cosmic vacuum cleaner. If our Sun was suddenly replaced with a black hole of the same mass, the Earth's orbit around the Sun would be unchanged. (Of course the Earth's temperature would change, and there would be no solar wind or solar magnetic storms affecting us.) To be "sucked" into a black hole, one has to cross inside the schwarzschild radius . At this radius, the escape speed is equal to the speed of light, and once light passes through, even it cannot escape.The Schwarzschild radius can be calculated using the equation for escape speed:
If We Can't See Them, How Do We Know They're There?

However, if a black hole passes through a cloud of interstellar matter , or is close to another "normal" star, the black hole can accerete matter into itself. As the matter falls or is pulled towards the black hole, it gains kinetic energy, heats up and is squeezed by tidal forces. The heating ionizes of ions , and when the atoms reach a few million kelvin, they emit X-rays . The X-rays are sent off into space before the matter crosses the Schwarzschild radius and crashes into the singulatrity. Thus we can see this X-ray emission.
Binary X-ray sources are also places to find strong black hole candidates. A companion star is a perfect source of infalling material for a black hole. A binary system also allows the calculation of the black hole candidate's mass. Once the mass is found, it can be determined if the candidate is a neutron star or a black hole, since neutron stars always have masses of about 1.5 times the mass of the Sun. Another sign of the presence of a black hole is its random variation of emitted X-rays. The infalling matter that emits X-rays does not fall into the black hole at a steady rate, but rather more sporadically, which causes an observable variation in X-ray intensity. Additionally, if the X-ray source is in a binary system, and we see it from certain angles, the X-rays will be periodically cut off as the source is eclisped by the companion star. When looking for black hole candidates, all these things are taken into account. Many X-ray satellite have scanned the skies for X-ray sources that might be black hole candidates.
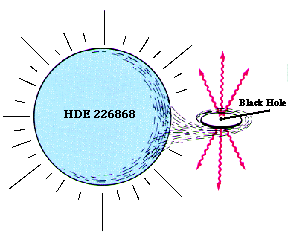
Cygnus X-1 (Cyg X-1) is the longest known of the black hole candidates. It is a highly variable and irregular source, with X-ray emission that flickers in hundredths of a second. An object cannot flicker faster than the time required for light to travel across the object. In a hundredth of a second , light travels 3,000 kilometers. This is one fourth of Earth's diameter! So the region emitting the X-rays around Cyg X-1 is rather small. Its companion star, HDE 226868 is a B0 supergiant with a surface temperature of about 31,000 K. spectroscopic observations show that the spectral line of HDE 226868 shift back and forth with a period of 5.6 days. From the mass-luminosityrelation, the mass of this supergiant is calculated as 30 times the mass of the Sun. Cyg X-1 must have a mass of about 7 solar masses, or else it would not exert enough gravitational pull to cause the wobble in the spectral lines of HDE 226868. Since 7 solar massis too large to be a white Dwarf or neutron star, it must be a black hole.
Nonetheless, there are now about 20 binaries (as of early 2009) for which the evidence for a black hole is much stronger than in Cyg X-1. The first of these, an X-ray transient called A0620-00, was discovered in 1975, and the mass of the compact object was determined in the mid-1980's to be greater than 3.5 solar masses. This very clearly excludes a neutron star, which has a mass near 1.5 solar masses, even allowing for all known theoretical uncertainties. The best case for a black hole is probably V404 Cygni, whose compact star is at least 10 solar masses. With improved instrumentation, the pace of discovery has accelerated, and the list of dynamically confirmed black hole binaries is growing rapidly.
What About All the Wormhole Stuff?
Unfortunately, wormholes are more science fiction than they are science fact. A wormhole is a theoretical opening in space-time that one could use to travel to far away places very quickly. The wormhole itself is two copies of the black hole geometry connected by a throat. The throat, or passageway, is called an Einstein-Rosen bridge. It has never been proven that wormholes exist, and there is no experimental evidence for them, but it is fun to think about the possibilities their existence might create.How an alternate theory of the universe exposes the war of words that underlies modern cosmology.
Theoretical physicists have recently been frustrated by a bold hypothesis concerning black holes—specifically, that they don’t exist.
In March, at the 22nd Pacific Coast Gravity Meeting in Santa Barbara, Calif., George Chapline, an applied physicist at Lawrence Livermore National Laboratory, gave a talk based on ideas he’s been incubating for several years. His goal: to amend astrophysics by applying theories of dark energy and condensed matter physics.
His work reinvents black holes as so-called “dark energy stars,” which are what is left over when matter transitions to dark energy as it passes a point of no return similar to a black hole’s event horizon. That redefinition, if correct, would invalidate much of the intellectual framework of traditional black holes.
Chapline’s ideas take inspiration from his colleague Robert Laughlin, a condensed matter physicist at Stanford University who won a Nobel for his work on quantum fluids.
Laughlin is quick to point out that the hubbub he and Chapline’s ideas have caused “is a battle of words rather than a battle of science.
“In science, you decide whose theory is right (or wrong) by means of an experiment,” he said, “not by polling experts.”
“Who wouldn’t want to be the researcher who dismantles Einstein and Hawking?”
Unfortunately for theoretical physicists, experimenting on the nature of the universe is not an easy undertaking. Revisionism of one sort or another is constantly occurring, due to the field’s heavier-than-normal reliance on theories based on observation, extrapolation and imagination.
“In some ways our playground is too big,” said Leonard Susskind, a theoretical particle physicist at Stanford and an outspoken critic of the Chapline-Laughlin theory.
“Practically speaking, much of our subject matter is inaccessible to direct experimentation,” he continued. “It doesn’t make the science any less valid—we didn’t need to go to the Moon to know that it wasn’t made of cheese.”
But indirection, inference and, ultimately, guesswork all chafe against some of science’s core values. Understandably, some researchers inevitably suggest less-fuzzy alternatives, which is how Chapline and Laughlin see their work.
“George and I made a very plausible case that general relativity, as we have observed it experimentally, could be perfectly true, and yet fail to describe a black hole event horizon properly,” said Laughlin. “What would allow this to happen is failure of the relativity principle on very short-length scales.”
His and Chapline’s model, he argues, fixes violations of quantum mechanics—such as information loss and the freezing of time at a black hole’s event horizon— in traditional black hole models. Laughlin notes that the argument may offend his peers, but that they have no valid criticism of his and his partner’s arguments. He insists their redefinition is correct.
“The point is that there is no way to tell one way or the other right now,” he said. “If there were, there would be no controversy.”
The Chapline-Laughlin hypothesis will linger like most cosmological theories, which are only partially or indirectly testable as well as often incomplete and replete with corrections needed to describe the universe we actually observe. The process of pinning on these amendments can get messy.
“This is starting to bug a lot of people,” said Geoff Marcy, an astronomer at the University of California at Berkeley. “You can end up with a patchwork that’s so ad hoc, with so many after-the-fact add-ons and addenda and caveats, that you might as well throw the whole thing out.”
Chapline and Laughlin face an uphill battle among the many theoretical physicists who have already devised their own fixes for the quantum violations of black holes either via string theory or a concept called “black hole evaporation,” wherein two particles fluctuate at the event horizon of a black hole so that one is sucked in while the other is shot out, making it seem as though the black hole is emitting the particle, or “evaporating.”
Samir Mathur, a physicist at the Ohio State University who has his own theories of black holes, which he calls “fuzzballs,” has no use for the Chapline-Laughlin theory.
“I feel comfortable dismissing it,” he said. “Their model does not account for the entropy of black holes, or for Hawking radiation. These are basic signatures of what black holes are. It appears that what is most appealing to them about their theory is that they are the ones who thought of it.”
For his part, Chapline suggests his critics are predictably lashing out at him using what he calls “the first law of physics,” where an idea is immediately derided if it questions well-ingrained notions.
“Experts don’t like it when you tell them they are not experts anymore, that books they have written are obsolete,” he said. “They don’t like to have to learn new things.”
Luboš Motl, a theoretical physicist at Harvard University, doesn’t buy the idea that black holes don’t exist. In fact, at Harvard, a NASA/Smithsonian partnership using the Chandra X-Ray Observatory has produced swarms of black hole data.
“Who wouldn’t want to be the researcher who dismantles Einstein and Hawking?” Motl said. “That is seductive. But this is a matter of ego, not science.”
Looking for Hawking Radiation in space is likely impossible with our current technology. But scientists here on Earth recently used flowing water to simulate a black hole and create event horizons, testing Stephen Hawking’s famous prediction that the event horizon creates particles and anti-particles.
Black holes resemble cosmic drains where space disappears like water draining out of a sink. Space seems to flow, and the closer one gets to the black hole, the faster it flows. At the event horizon, space appears to reach the speed of light, so nothing, not even light, can escape beyond this point of no return.
Researchers from the University of St. Andrews and the University of Nice used a water channel to create analogues of black holes, simulating event horizons.
The scientists sent waves against the current, varied the water speed and the wavelength, and filmed the waves with video

They used a 30-meter-long water channel with a powerful pump on one end and a wave machine on the other, which is normally used to test the environmental impact of currents and waves on coasts or the hulls of submarines.
While the water didn’t create anti-particles, the researchers may have seen “anti-waves.” Normal waves heave up and down in the direction they move, whereas anti-waves do the opposite.
One of the researchers, Professor Ulf Leonhardt said, “It is probably impossible to observe the Hawking radiation of black holes in space, but something like the radiation of black holes can be seen on Earth, even in something as simple as flowing water.”
“We definitely have observed these negative-frequency waves. These waves were tiny, but they were still significantly stronger than expected. However, our experiment does not completely agree with theory and so much work remains to be done to understand exactly what happens at the event horizon for water waves.”
Their research will be published in the New Journal of Physics.
What is "causality" and what does it have to do with time travel?
What do we mean by time travel?
 Technically we're all travelling in time just by existing. But we can't seem to control our motion through time in the same way we can control our motion through space. What most people usually mean by time travel is the ability to drive around in time they same way they'd drive around in a city.
Technically we're all travelling in time just by existing. But we can't seem to control our motion through time in the same way we can control our motion through space. What most people usually mean by time travel is the ability to drive around in time they same way they'd drive around in a city.  Let's look at a spacetime diagram that shows a few examples of different ways observers or objects can travel in time and space.
Let's look at a spacetime diagram that shows a few examples of different ways observers or objects can travel in time and space.  It's important to remember that any single point on this diagram represents an event - both a moment in time and a location in space. The origin of the axes in the figure represents the place X=0 at the time T=0. This is what we mean by a spacetime event.
It's important to remember that any single point on this diagram represents an event - both a moment in time and a location in space. The origin of the axes in the figure represents the place X=0 at the time T=0. This is what we mean by a spacetime event.  The figure to the left is a spacetime diagram showing spacetime paths of various observers moving in 1+1 dimensional spacetime. Paths A, B, C and D represent the normal kind of time travel that we find in our world. Path E shows an example of a kind of time travel that is not allowed in Special Relativity.
The figure to the left is a spacetime diagram showing spacetime paths of various observers moving in 1+1 dimensional spacetime. Paths A, B, C and D represent the normal kind of time travel that we find in our world. Path E shows an example of a kind of time travel that is not allowed in Special Relativity. The allowed time travellers
 The light blue spacetime path A represents a flash of light from a laser coming from off of the diagram. The flash of light travels into the future to the spacetime event T=0, X=0 at which it intersects with the spacetime path B.
The light blue spacetime path A represents a flash of light from a laser coming from off of the diagram. The flash of light travels into the future to the spacetime event T=0, X=0 at which it intersects with the spacetime path B.  Path B is a vertical line. A vertical line on this diagram means an observer or object at rest in this coordinate system, staying at the same value of X for all time T. In this example, the green spacetime path B is the worldline of a mirror standing on a table. Let's say that this mirror is only half-silvered, so that when the laser pulse path A intersects with it, half of the pulse is reflected back to the source.
Path B is a vertical line. A vertical line on this diagram means an observer or object at rest in this coordinate system, staying at the same value of X for all time T. In this example, the green spacetime path B is the worldline of a mirror standing on a table. Let's say that this mirror is only half-silvered, so that when the laser pulse path A intersects with it, half of the pulse is reflected back to the source.  The reflected half of the laser pulse is shown by the light blue spacetime path C. The half of the pulse that is not reflected but transmitted is found by continuing path A.
The reflected half of the laser pulse is shown by the light blue spacetime path C. The half of the pulse that is not reflected but transmitted is found by continuing path A.  The purple spacetime path D starts at rest, then accelerates and keeps accelerating until it approaches the speed of light.
The purple spacetime path D starts at rest, then accelerates and keeps accelerating until it approaches the speed of light. The forbidden time travellers
 Our abnormal time traveller is shown on the red spacetime path E. Notice this path is a circle. But it isn't like a circle in space -- anyone can walk around a circle in space. This path is a circle in spaceTIME -- this path keeps going back to the same TIME as well as back to the same space.
Our abnormal time traveller is shown on the red spacetime path E. Notice this path is a circle. But it isn't like a circle in space -- anyone can walk around a circle in space. This path is a circle in spaceTIME -- this path keeps going back to the same TIME as well as back to the same space.  Notice also that spacetime path E is almost always in two places at the same time.
Notice also that spacetime path E is almost always in two places at the same time.  What's wrong with this spacetime path?
What's wrong with this spacetime path?  Over 50% of spacetime path E is travelling faster than the speed of light. At the top and bottom of the circle, the observer would have to be travelling infinitely fast.
Over 50% of spacetime path E is travelling faster than the speed of light. At the top and bottom of the circle, the observer would have to be travelling infinitely fast.  We'll explore the difference between normal and abnormal time travellers in the next section.
We'll explore the difference between normal and abnormal time travellers in the next section.
How does the Special Theory of Relativity work?
The Lorentz transformation Recall that in the previous section, we tried to model space and time in a way that would be consistent with the observed constancy of the speed of light in Nature. The resulting model for space and time measurements has observers measuring time and space differently when they are moving relatively to one another. The two basic differences between what relatively-moving observers measure can be summarized as: Recall that in the previous section, we tried to model space and time in a way that would be consistent with the observed constancy of the speed of light in Nature. The resulting model for space and time measurements has observers measuring time and space differently when they are moving relatively to one another. The two basic differences between what relatively-moving observers measure can be summarized as:
 We will now state (without going through the grunge work of proving) that the mathematical model that best encompasses the observed constancy of the speed of light in Nature (pretending for now that gravity doesn't exist) is the one where the spacetime coordinates of two such observers as described above are related through what is now called a Lorentz transformation: We will now state (without going through the grunge work of proving) that the mathematical model that best encompasses the observed constancy of the speed of light in Nature (pretending for now that gravity doesn't exist) is the one where the spacetime coordinates of two such observers as described above are related through what is now called a Lorentz transformation:
 This transformation tells us how some observer O, who sees another observer O' moving at velocity U, translates measurements made by O' into measurements valid in the O frame of reference. In our specific case, we'd say this is how the red driver sees things that are happening in the frame of reference of the blue driver. This transformation tells us how some observer O, who sees another observer O' moving at velocity U, translates measurements made by O' into measurements valid in the O frame of reference. In our specific case, we'd say this is how the red driver sees things that are happening in the frame of reference of the blue driver.  For example, in time dilation: in the blue car's frame of reference, the laser pulse did not travel in the Xb-direction at all, so X' = Xb = 0, leaving T = T' /(1 - U2/c2)1/2. For example, in time dilation: in the blue car's frame of reference, the laser pulse did not travel in the Xb-direction at all, so X' = Xb = 0, leaving T = T' /(1 - U2/c2)1/2.  In length contraction: in the red car's frame of reference, the red driver must measure the length of the blue car at a single moment of the red driver's time. This means we have Tr = T = 0 and so c T' = - (U/c) X', leading to X = X' (1 - U2/c2)1/2. In length contraction: in the red car's frame of reference, the red driver must measure the length of the blue car at a single moment of the red driver's time. This means we have Tr = T = 0 and so c T' = - (U/c) X', leading to X = X' (1 - U2/c2)1/2.  The Lorentz transformation is the foundation of relativistic geometry, which we will examine next. The Lorentz transformation is the foundation of relativistic geometry, which we will examine next. |
Why was the Special Theory of Relativity needed?
The velocity addition problem
 The observed constancy of the speed at which light travels tells us that the Newtonian model of space and time is flawed. But the flaws don't become noticeable until we start trying to describe things moving near the speed of light.
The observed constancy of the speed at which light travels tells us that the Newtonian model of space and time is flawed. But the flaws don't become noticeable until we start trying to describe things moving near the speed of light. 
 One reason why Einstein's Special Theory of Relativity was needed was because of the special problems that cropped up when scientists noticed that the speed of light is a constant everywhere in every direction. This caused problems with the Newtonian model for measuring time. One of these problems is called the velocity addition problem.
One reason why Einstein's Special Theory of Relativity was needed was because of the special problems that cropped up when scientists noticed that the speed of light is a constant everywhere in every direction. This caused problems with the Newtonian model for measuring time. One of these problems is called the velocity addition problem.  The velocity addition problem is illustrated in the figures above and below. In the top figure we see the red driver's frame of reference or rest frame. To the driver of the red car in her rest frame, the blue car is travelling forward at velocity U and some purple object is flying out of the blue car at velocity V.
The velocity addition problem is illustrated in the figures above and below. In the top figure we see the red driver's frame of reference or rest frame. To the driver of the red car in her rest frame, the blue car is travelling forward at velocity U and some purple object is flying out of the blue car at velocity V.  In the figure below the point of view of the blue driver is illustrated. To the driver of the blue car, the purple object is flying forwards out of her window at velocity V', and the red car is driving backwards.
In the figure below the point of view of the blue driver is illustrated. To the driver of the blue car, the purple object is flying forwards out of her window at velocity V', and the red car is driving backwards. 
 The velocity addition problem asks the question:
The velocity addition problem asks the question:  Given U and V', what is V?
Given U and V', what is V?  If we use Newton's model for time as being experienced exactly the same for all observers, we wind up with the answer: V = U + V'. How?
If we use Newton's model for time as being experienced exactly the same for all observers, we wind up with the answer: V = U + V'. How?  Suppose, as Newton believed, the red and blue drivers measure time and distance precisely the same. According to the blue driver, in time T the distance of the purple ball from the blue car is Xball = V' T. In the same time T in the reference frame of the red car, the blue car has travelled the distance Xcar = U T. According to the red driver, the total distance the purple ball travelled is the distance the blue car travelled from the red car plus the distance the purple ball travelled from the blue car, or X = Xcar + Xball = U T + V' T = (U + V') T.
Suppose, as Newton believed, the red and blue drivers measure time and distance precisely the same. According to the blue driver, in time T the distance of the purple ball from the blue car is Xball = V' T. In the same time T in the reference frame of the red car, the blue car has travelled the distance Xcar = U T. According to the red driver, the total distance the purple ball travelled is the distance the blue car travelled from the red car plus the distance the purple ball travelled from the blue car, or X = Xcar + Xball = U T + V' T = (U + V') T.  But X = V T, and that gives us the velocity addition formula:
But X = V T, and that gives us the velocity addition formula: V = U + V'
 So, for example, if the blue car is going 30 mph and the driver of the blue car measures the purple ball going 60 mph, then the driver of the red car should measure the purple ball going 90 mph, because U = 30 mph, V' = 60 mph and hence V = U + V' = 90 mph.
So, for example, if the blue car is going 30 mph and the driver of the blue car measures the purple ball going 60 mph, then the driver of the red car should measure the purple ball going 90 mph, because U = 30 mph, V' = 60 mph and hence V = U + V' = 90 mph.  Sounds reasonable, right?
Sounds reasonable, right?  Okay, now suppose that U = half the speed of light, and V' = the speed of light (maybe the purple object is a laser pulse). Now what is V? The above formula tells us V = one and a half times the speed of light.
Okay, now suppose that U = half the speed of light, and V' = the speed of light (maybe the purple object is a laser pulse). Now what is V? The above formula tells us V = one and a half times the speed of light.  This is contrary to observed behavior of Nature. Therefore the Newtonian model of time as being experienced equally by all observers must not be a good model for Nature.
This is contrary to observed behavior of Nature. Therefore the Newtonian model of time as being experienced equally by all observers must not be a good model for Nature.
SPACE TIME
Geometry is "measuring the Earth"
 How did human beings come to use mathematics to describe the world around them? One of the early motivators for humans to perfect a language for communicating about the world in terms of numbers came from the need to measure the Earth. People were learning to build large temples and cultivate large fields. These people had spiritual and practical needs for understanding how to measure and describe the space around them.
How did human beings come to use mathematics to describe the world around them? One of the early motivators for humans to perfect a language for communicating about the world in terms of numbers came from the need to measure the Earth. People were learning to build large temples and cultivate large fields. These people had spiritual and practical needs for understanding how to measure and describe the space around them.  The word geometry reflects this need. Geo is Greek for Earth, from the very ancient Greek Earth Goddess Gaia. Meter is related to measure and also to mother. But although the ancient Greeks succeeded in naming most of geometry, they were not the first people to discover much of what they've been given credit for. The ancient Mesopotamians figured out much of what the Greeks wrote down a millenium later, including what became known as the Pythagorean Rule:
The word geometry reflects this need. Geo is Greek for Earth, from the very ancient Greek Earth Goddess Gaia. Meter is related to measure and also to mother. But although the ancient Greeks succeeded in naming most of geometry, they were not the first people to discover much of what they've been given credit for. The ancient Mesopotamians figured out much of what the Greeks wrote down a millenium later, including what became known as the Pythagorean Rule: 
L12 + L22 = LH2where L1 and L2 are the lengths of the two legs of the right triangle shown in the figure, and LH is the length of the hypotenuse of that right triangle.
 The Mesopotamians discovered this rule by observation, not by formal derivation from abstract mathematical priciples. They measured things like clay tablets and fields of wheat. They were discovering something important about mathematics and Nature simultaneously.
The Mesopotamians discovered this rule by observation, not by formal derivation from abstract mathematical priciples. They measured things like clay tablets and fields of wheat. They were discovering something important about mathematics and Nature simultaneously.  As far as we know, it wasn't until ancient Greece that a system of abstract principles describing geometry emerged.
As far as we know, it wasn't until ancient Greece that a system of abstract principles describing geometry emerged.  The Pythagorean Rule became a theorem provable from completely abstract arguments (based on a few key assumptions that, as we shall see later, Nature doesn't actually respect), independent from observations made by measuring things. This development marked what we now know as Euclidean geometry, named after the Greek mathematician Euclid who wrote the first known geometry book, known today as Euclid's Elements, which gathered together the accumulated understanding of his time.
The Pythagorean Rule became a theorem provable from completely abstract arguments (based on a few key assumptions that, as we shall see later, Nature doesn't actually respect), independent from observations made by measuring things. This development marked what we now know as Euclidean geometry, named after the Greek mathematician Euclid who wrote the first known geometry book, known today as Euclid's Elements, which gathered together the accumulated understanding of his time. Who was Pythagoras?
 The Pythagorean Rule was not described by Pythagoras himself. Pythagoras is remembered for having observed and eloquently described (for his time) the numerical relationships between musical tone scales and the length scales of the physical objects producing them, such as the lengths of the strings on stringed instruments and the diameters of bells.
The Pythagorean Rule was not described by Pythagoras himself. Pythagoras is remembered for having observed and eloquently described (for his time) the numerical relationships between musical tone scales and the length scales of the physical objects producing them, such as the lengths of the strings on stringed instruments and the diameters of bells.  The Pythagorean Rule itself probably came from followers of his school of philosophy, whom we call Pythagoreans.
The Pythagorean Rule itself probably came from followers of his school of philosophy, whom we call Pythagoreans.
Subscribe to:
Posts (Atom)





